At a recent book launch and signing for Philip Ording’s 99 Variations on a Proof (Princeton, New Jersey: Princeton University Press, 2019. QA8.4 .O73 2019), mathematician Ording kindly left a note in our copy for Princeton students. The event was hosted in the Ludlow Street basement studio of David Reinfurt, Princeton Lecturer in Visual Arts, graciously overseen by several former students.

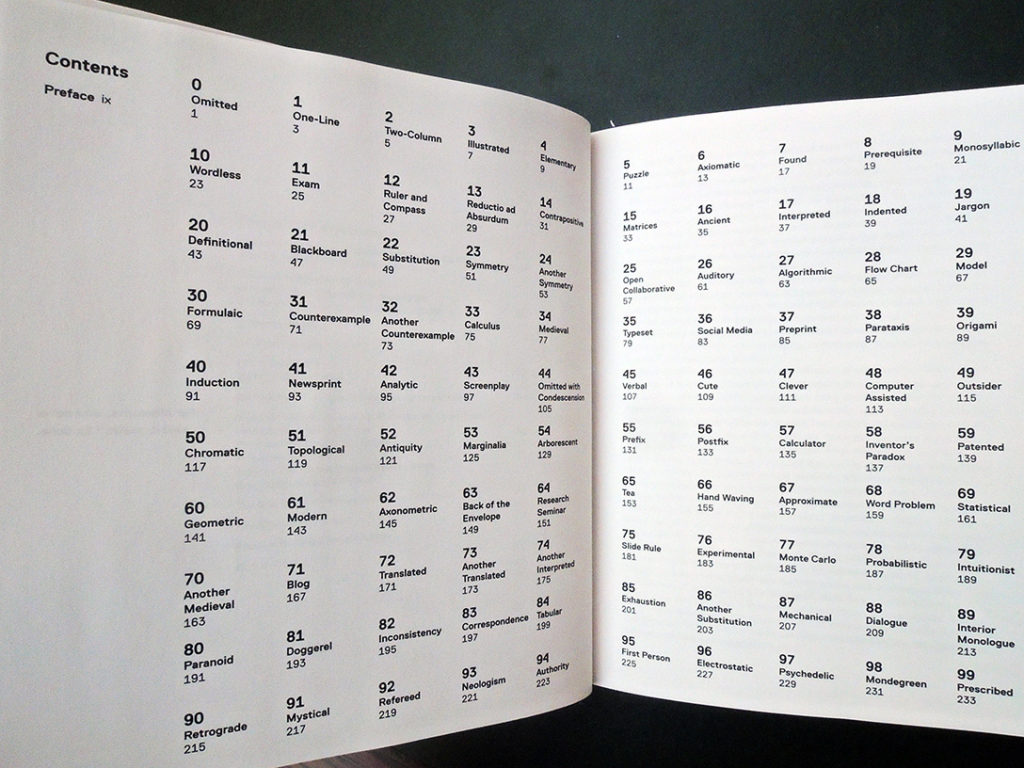
“This book offers a multifaceted perspective on mathematics by demonstrating 99 different proofs of the same theorem. Each chapter solves an otherwise unremarkable equation in distinct historical, formal, and imaginative styles that range from Medieval, Topological, and Doggerel to Chromatic, Electrostatic, and Psychedelic [note the reference to Princeton in this chapter].

“…Inspired by the experiments of the Paris-based writing group known as the Oulipo – whose members included Raymond Queneau, Italo Calvino, and Marcel Duchamp – Ording explores new ways to examine the aesthetic possibilities of mathematical activity. 99 Variations on a Proof is a mathematical take on Queneau’s Exercises in Style, a collection of 99 retellings of the same story, and it draws unexpected connections to everything from mysticism and technology to architecture and sign language.”–Back cover.

The cubic equation in question and claim is: If x³−6x²+11x−6=2x−2, then x=1 or x=4.

“Fun, funny, and unexpectedly deep, Philip Ording’s Oulipian expedition through the far reaches of mathematical style shows there’s more than one way to skin a cubic equation.”—Jordan Ellenberg, author of How Not to Be Wrong: The Power of Mathematical Thinking https://press.princeton.edu/titles/13308.html

Philip Ording is a professor of mathematics at Sarah Lawrence College. He is the coeditor of Simplicity: Ideals of Practice in Mathematics and the Arts.